High School Math Competition
2020-01-08
1 Circle
1.1 Basics
- AB is a diameter of circle \(O\), \(PA\) is a tangent line of the circle. \(C\) is ther intersect point of \(PO\) and the circle. \(\angle P=40^o\). What’s \(\angle B\)?
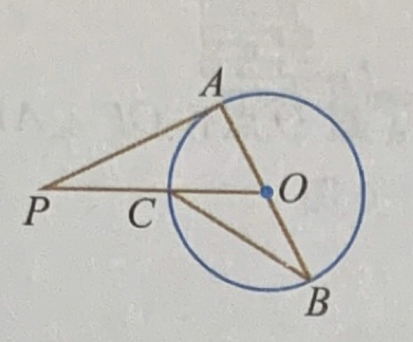
Figure 1.1: Circle 1
- The two legs of a right \(\triangle ABC\) is tagent with a circle \(O\) whose center \(O\) is the mid point of the hypotenuse of \(\triangle ABC\). D and E are the tagent points on \(AB\) and \(AC\). \(BC = 2\sqrt 2\). What’s the length of arc \({DE}\)?
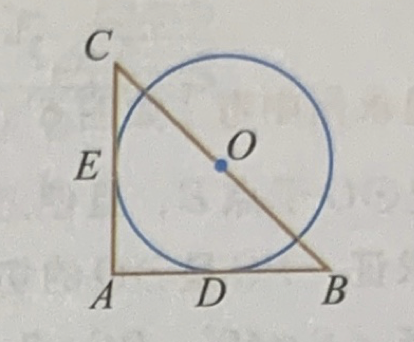
Figure 1.2: Circle 2
A circle \(O\) has radius 10, AB and CD are two chords of the circle. \(AB \parallel BC\), \(AB = 12\), \(CD=16\), what’s the distance between AB and CD?
Triangle \(ABC\), \(AB=AC\), \(\angle ABC = 30^o\). \(BD\) is a diameter of \(\triangle ABC\)’s circumsribe circle, \(CD=\frac{4\sqrt 3}{3}\), AD=?
\(AB\) is a chord of circle \(O\). \(E\) is the midpoint of \(AB\). \(EC\perp OA\), BD is a tangent line of Circle \(O\). \(AB=12\), \(BD=5\). What is the radius of the circle?
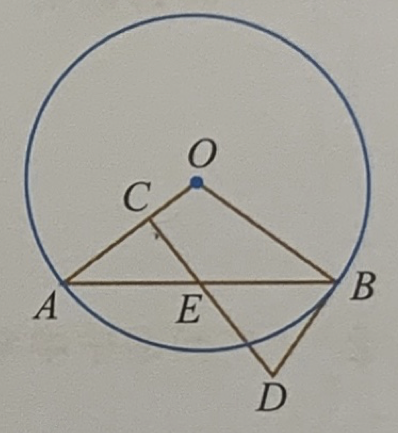
Figure 1.3: Circle 5
- BC is the diameter of circle O with radius 3. Circle O interset with AC at point E. EF is a tangent line of Circle O and \(EF \perp AB\) at point F. EF is extended and intersect with CB’s extended line at G,\(\angle ABG=2\angle C\), \(\sin \angle EGC = \frac{3}{5}\). AF=?
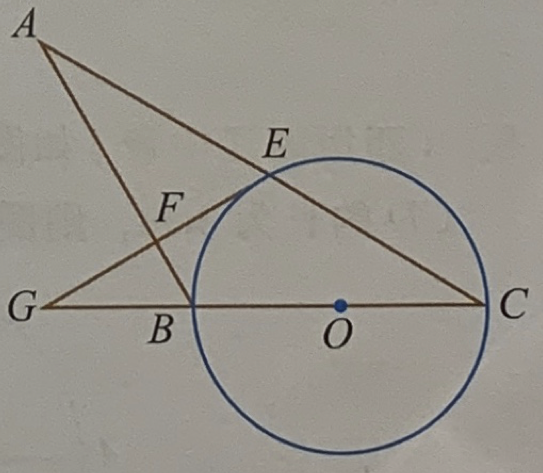
Figure 1.4: Circle 6