7.3 Digital Imaging

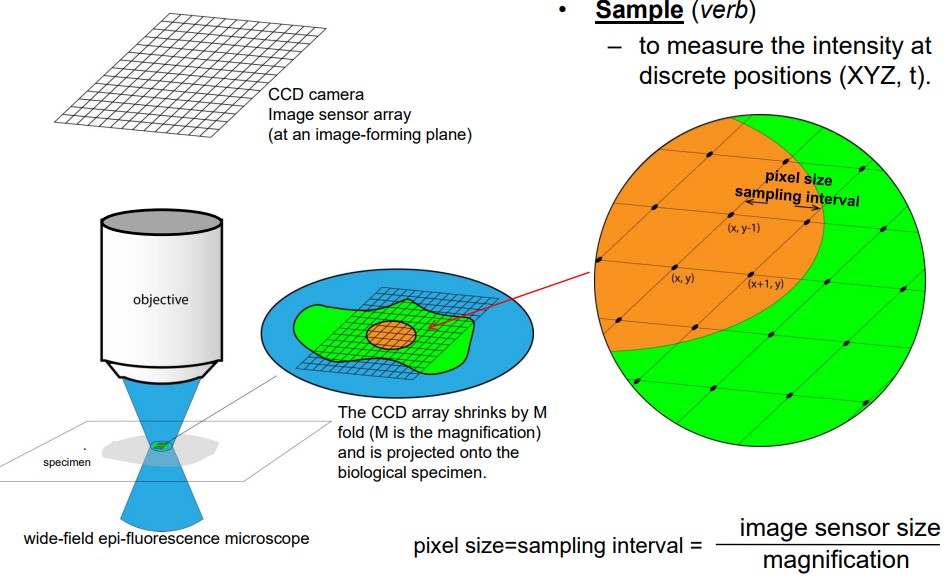
Figure 7.15: Visual Explanation of Digital Imaging

Digital imaging is a process of sampling using image sensors. The magnification controls the sampling intervals.

7.3.1 Nyquist Sampling Theorem

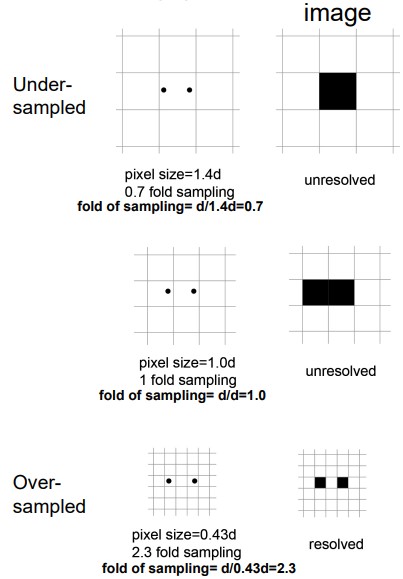
Figure 7.16: Simplified Explanation of Nyquist Sampling Theorem

To fully utilize a microscope system’s resolution, the image has to be sampled at the Nyquist rate.

The sampling interval should be less than half of the resolution distance or feature. Two to three folds of sampling is typically required.

7.3.1.1 Nyquist Sampling

The pixel size should be less than or equal to the resolution distance. For an objective lens with NA1.4, its resolution is about 200 nm. Hence, the largest pixel size should be about 100 nm.

Hence, to image a square region of 20 by 20 micrometers, the minimal scanning resolution is \(\displaystyle \frac{20 \text{ }\mu m}{100\text{ nm}} = 200\). This means that a scanning resolution that is greater than or equal to 200 by 200 should be utilized.

7.3.2 Basic Image Manipulation

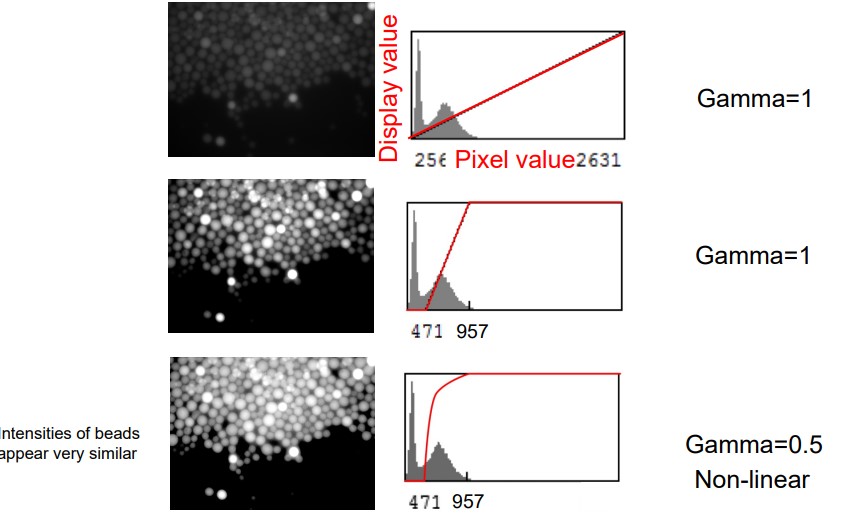
Figure 7.17: Difference Between Brightness, Gamma, and Contrast

Brightness, contrast, and gamma changes the display, but not the value of the image.

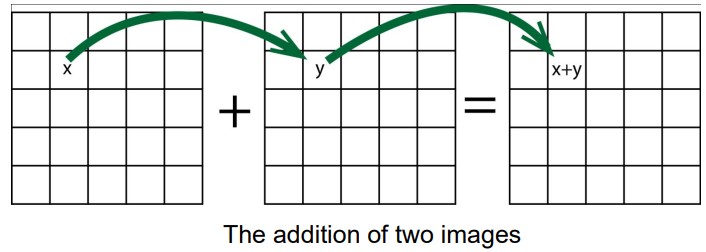
Figure 7.18: Image Arithmeitc Illustrated

Image arithmetic is a point-wise operation. The two images need to have the same dimension.

Figure 7.19: How Signal-to-Noise Ratio Can be Improved

The SNR is an acronym for signal to noise ratio.

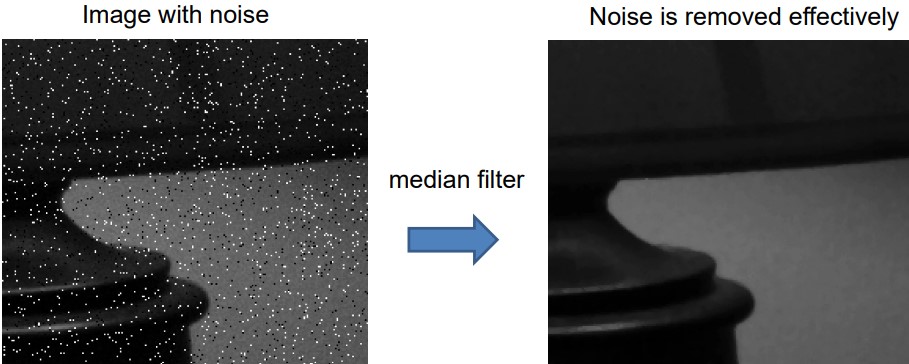
Figure 7.20: Filtering

Filtering is an operation to compute the new value of a pixel using its neighboring pixels.